n with X=A,B,C,D,E,F or G denotes the affine WZNW model of level k based on the simple Lie-algebra Xn of rank n;
Vir(n,k) with coprime integers n and k, denotes the minimal Virasoro model of central charge c=1-6
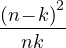 ;
;
of radius R= ), i.e. the coset
), i.e. the coset 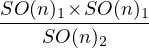 ;
;
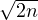 ), i.e.
), i.e. the U(1) WZNW model at level n;
SVir1(n) denotes the minimal N =1 superconformal model of central charge c =
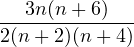 , i.e. the
, i.e. the
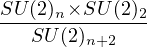 coset model;
coset model;
SVir2(n) denotes the minimal N=2 superconformal model of central charge c= 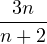 , i.e. the
, i.e. the
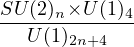
coset model;
PF(n) denotes the parafermionic model of central charge c=
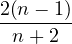 , i.e. the
, i.e. the 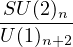 coset;
coset;
respectively the cyclic, dihedral, symmetric, alternating and Mathieu groups of degree n;
T, O and I denote the isolated c= 1 models, i.e. the orbifolds of SU(2)1 with tetrahedral, octahedral and
icosahedral respective twist groups.
Diagrams
The following table summarizes some of the characteristics of locality diagrams, ordered by
increasing number of vertices. The first column gives the conventional label of the diagram, the second
the total number Υ of vertices, the third the number ϵ of essential ones, the fourth the size 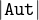 of the
automorphism group (when known), the fifth the number ρ of loops (self-adjacent vertices), the sixth
the size λ of the associated lattice, the seventh the dimension δ (length of a maximal chain) of the
latter, and finally, the eighth lists some conformal models with the given locality diagram.
of the
automorphism group (when known), the fifth the number ρ of loops (self-adjacent vertices), the sixth
the size λ of the associated lattice, the seventh the dimension δ (length of a maximal chain) of the
latter, and finally, the eighth lists some conformal models with the given locality diagram.
| label | Υ | ϵ | 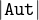 | ρ | λ | δ | examples |
|
2 |
1 |
1 |
1 |
2 |
1 | G(1), A
1 1, A 1 2, A 1 4, A 1 6, E 1 6, E 1 7, |
|
| E
n+2 8 (n<5), F n 4 (n<9), G n 2 (n<9) |
|||||||
|
3 |
2 |
1 |
2 |
3 |
2 | D(M11), PF(2), A
2 1, A 1 3, A 1 8, B 1 n, |
|
| C
1 2, D 1 2n+3, E 2 8, G(2), Vir(4,3) |
|||||||
| 59A | 4 | 2 | 2 | 1 | 4 | 2 | PF(p), A
2n+1 1, A 3n∓1 2, G(p) |
| 9A | 4 | 3 | 1 | 2 | 4 | 3 | AT(1), A
2n 1, A 3 2, C 1 2n+2, G(4) |
| 62A | 4 | 3 | 6 | 4 | 5 | 2 | D(ℤ2), D
1 4n (n ≤ 3) |
| 62B | 4 | 3 | 2 | 2 | 5 | 2 | D
1 4n+2 (n ≤ 3) |
| 84A | 5 | 4 | 1 | 3 | 5 | 4 | D(𝕊5), D(𝕊6), D(M10), B
2 4, G(8) |
| 76A | 5 | 4 | 4 | 3 | 6 | 2 | D(ℤ3) |
| 76B | 5 | 4 | 8 | 1 | 6 | 2 | |
|
6 |
3 |
1 |
2 |
6 |
3 | PF(p2), A
2n+1 3, A 2 8, A 4 8, D 3 5, D 3 7, D 3 9, E 2 7 |
|
G(2p), G(p2), SVir2(1), SVir1(3)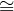 Vir(5,4) Vir(5,4) |
|||||||
| 11A | 6 | 4 | 2 | 2 | 6 | 4 | PF(4),AT(p), A
2 3, A 3 8, D 2 5, D 2 7, D 2 11, D 2 13 |
| 61A | 6 | 5 | 1 | 3 | 6 | 5 | A
4 3, D 4 5, G(16), T |
| 186A | 6 | 5 | 2 | 2 | 8 | 3 | |
| 82A | 7 | 6 | 4 | 4 | 8 | 4 | D(𝕊3) |
| 93A | 7 | 6 | 16 | 3 | 8 | 2 | D(ℤ5) |
| 93B | 7 | 6 | 48 | 1 | 8 | 2 | |
| 172A | 7 | 6 | 1 | 4 | 7 | 6 | G(32) |
| 71A | 8 | 3 | 6 | 1 | 8 | 3 | PF(15), A
5 5, A 7 5, G(pq) |
| 63A | 8 | 4 | 6 | 4 | 10 | 3 | D
3 4, D 5 4, D 3 8 |
| 63B | 8 | 4 | 2 | 2 | 10 | 3 | D
3 6, D 3 10 |
|
8 |
4 |
1 |
2 |
8 |
4 | A
2 5, A 3 5, A 4 5, A 8 5, A 5 7, Vir(n+5,n+4), |
|
| PF(2p), G(4p), G(p3), PF(27) | |||||||
| 90A | 8 | 5 | 2 | 4 | 8 | 5 | A
6 5 |
| 15A | 8 | 5 | 1 | 3 | 8 | 5 | PF(8),AT(p2), A
2 7, D 2 9 |
| 118A | 8 | 7 | 12 | 5 | 9 | 4 | D(𝔸4) |
| 91A | 8 | 6 | 2 | 3 | 8 | 6 | A
4 7 |
| 12A | 8 | 7 | 6 | 4 | 10 | 5 | AT(4), D
2 4, D 4 4, D 6 4, D 4 6, D 4 8 |
| 174A | 8 | 7 | 1 | 4 | 8 | 7 | G(64) |
| 187A | 8 | 7 | 6 | 2 | 10 | 3 | |
| 83A | 9 | 7 | 6 | 6 | 9 | 6 | D(𝕊4) |
| 10A | 9 | 8 | 4 | 4 | 13 | 4 | AT(2) |
| 95A | 9 | 8 | 96 | 3 | 10 | 2 | D(ℤ7) |
| 95B | 9 | 8 | 384 | 1 | 10 | 2 | |
| 14A | 9 | 8 | 6 | 5 | 11 | 6 | AT(8), D
2 8 |
| 170A | 9 | 4 | 2 | 4 | 9 | 4 | G(2p2) |
| 180A | 9 | 8 | 1 | 5 | 9 | 8 | G(128) |
| 184A | 9 | 8 | 16 | 4 | 10 | 4 | D(𝔻5) |
| 17A | 10 | 5 | 6 | 2 | 10 | 5 | AT(pq) |
| 21A | 10 | 6 | 1 | 3 | 10 | 6 | AT(27), PF(16) |
| 171A | 10 | 5 | 1 | 3 | 10 | 5 | G(8p) |
| 92A | 10 | 9 | 16 | 8 | 15 | 4 | D(ℤ4) |
| 92B | 10 | 9 | 48 | 4 | 15 | 4 | |
| 92C | 10 | 9 | 8 | 4 | 15 | 4 | |
| 92D | 10 | 9 | 8 | 6 | 15 | 4 | |
| 13A | 10 | 7 | 2 | 4 | 12 | 5 | AT(2p), D
2 6, D 2 10, SVir1(4) |
| 18A | 10 | 9 | 6 | 5 | 12 | 7 | AT(16) |
| 192A | 10 | 9 | 1 | 5 | 10 | 9 | G(28) |
| 23A | 11 | 10 | 6 | 6 | 13 | 8 | AT(32) |
| 193A | 11 | 10 | 1 | 6 | 11 | 10 | |
| 190A | 11 | 10 | 12 | 2 | 14 | 4 | |
| 190B | 11 | 10 | 12 | 4 | 14 | 4 | |
| 185A | 11 | 10 | 96 | 4 | 12 | 4 | D(𝔻7) |
|
12 |
4 |
2 |
2 |
12 |
4 | G(p2q), G(2pq), SVir2(p-2) for p > 3, | |
| SVir1(2n+1) | |||||||
| 70A | 12 | 5 | 2 | 2 | 12 | 5 | PF(12) |
| 72A | 12 | 5 | 1 | 4 | 12 | 5 | PF(18), G(4p2), G(2p3) |
| 31A | 12 | 7 | 1 | 4 | 12 | 7 | AT(81), PF(32) |
| 16A | 12 | 8 | 2 | 4 | 14 | 6 | AT(4p), D
2 12 |
| 29A | 12 | 11 | 6 | 6 | 14 | 9 | AT(64) |
| 4A | 12 | 11 | 2 | 4 | 18 | 5 | SVir2(2) |
| 173A | 12 | 6 | 1 | 3 | 12 | 6 | G(16p) |
| 188A | 12 | 11 | 120 | 2 | 14 | 3 | |
| 19A | 13 | 8 | 2 | 6 | 15 | 6 | AT(2p2) |
| 99A | 13 | 12 | 7680 | 3 | 14 | 2 | D(ℤ11) |
| 165A | 13 | 12 | 7 | 15 | 10 | AT(128) | |
| 25A | 14 | 6 | 2 | 3 | 14 | 6 | AT(p2q) |
| 121A | 14 | 8 | 1 | 4 | 14 | 8 | AT(243), PF(64) |
| 169A | 14 | 8 | 2 | 4 | 16 | 6 | SVir1(2n+2) |
| 20A | 14 | 9 | 2 | 5 | 16 | 7 | AT(8p) |
| 89A | 14 | 12 | 144 | 9 | 16 | 8 | D(M9) |
| 176A | 14 | 7 | 4 | 14 | 7 | G(32p) | |
| 101A | 15 | 14 | - | 3 | 16 | 2 | D(ℤ13) |
| 108A | 15 | 14 | - | 4 | 16 | 4 | D(𝔻11) |
| 175A | 15 | 6 | 6 | 15 | 6 | G(8p2), G(2p4) | |
| 77A | 16 | 15 | 16 | 67 | 4 | D(𝔻2) | |
| 124A | 16 | 5 | 2 | 16 | 5 | PF(30), G(4pq), G(135) | |
| 73A | 16 | 6 | 3 | 16 | 6 | PF(24) | |
| 22A | 16 | 8 | 4 | 18 | 6 | AT(2pq) | |
| 24A | 16 | 9 | 6 | 18 | 7 | AT(4p2) | |
| 26A | 16 | 10 | 5 | 18 | 8 | AT(16p) | |
| 27A | 16 | 9 | 6 | 18 | 7 | AT(54) | |
| 122A | 16 | 9 | 5 | 16 | 9 | AT(36) | |
| 177A | 16 | 4 | 1 | 16 | 4 | G(105) | |
| 178A | 16 | 6 | 4 | 16 | 6 | G(108) | |
| 110A | 17 | 16 | 4 | 18 | 4 | D(𝔻13) | |
| 97A | 17 | 16 | 96 | 7 | 23 | 4 | D(ℤ9) |
| 8A | 18 | 5 | 4 | 18 | 5 | G(2p2q), SVir2(2p2) | |
| 162A | 18 | 6 | 2 | 18 | 6 | AT(105) | |
| 168A | 18 | 6 | 4 | 18 | 6 | PF(36) | |
| 181A | 18 | 7 | 6 | 18 | 7 | G(144) | |
| 33A | 18 | 11 | 6 | 20 | 9 | AT(96) | |
Comments
- There is only one non-planar (reduced) diagram with less than 11 vertices - the one labelled 18A -, while for a larger number of vertices planar diagrams are the exception.
- Many of the diagrams - e.g., those of all Gaussian and parafermionic models - are extremal, i.e. they saturate the fundamental inequality δ ≤ ϵ< Υ ≤ λ in the sense that δ=ϵ and Υ=λ.
- Apart from a couple of degenerate cases, the structure of diagrams for unitary minimal Virasoro and N=1 superconformal models follows a simple pattern: there is just one generic Virasoro diagram (3A), while there are two generic N=1 superconformal diagrams (5A and 169A) depending on wether n is odd or even. For other classes of models, the pattern is more complicated, e.g. for N=2 superconformal and Ashkin-Teller models it depends on the prime factorization of the parameter n.